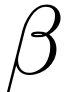1
2
3
4
5
6
7
8
9
10
11
12
13
14
15
16
17
18
19
20
21
22
23
24
25
26
27
28
29
30
31
32
33
34
35
36
37
38
39
40
41
42
43
44
45
46
47
48
49
50
51
52
53
54
55
56
57
58
59
60
61
62
63
64
65
66
67
68
69
70
71
72
73
74
75
76
77
78
79
80
81
82
83
84
85
86
87
88
89
90
91
92
93
94
95
96
97
98
99
100
101
102
103
104
105
106
107
108
109
110
111
112
113
114
115
116
117
118
119
120
121
122
123
124
125
126
127
128
129
130
131
132
133
134
135
136
137
138
139
140
141
142
143
144
145
146
147
148
149
150
151
152
153
154
155
156
157
158
159
160
161
162
163
164
165
166
167
168
169
170
171
172
173
174
175
176
177
178
179
180
181
182
183
184
185
186
187
188
189
190
191
192
193
194
195
196
197
198
199
|
/*
Copyright 2015-2022 Ian Jauslin
Licensed under the Apache License, Version 2.0 (the "License");
you may not use this file except in compliance with the License.
You may obtain a copy of the License at
http://www.apache.org/licenses/LICENSE-2.0
Unless required by applicable law or agreed to in writing, software
distributed under the License is distributed on an "AS IS" BASIS,
WITHOUT WARRANTIES OR CONDITIONS OF ANY KIND, either express or implied.
See the License for the specific language governing permissions and
limitations under the License.
*/
#ifdef RATIONAL_AS_FLOAT
#include "rational_float.h"
#include <stdio.h>
#include <stdlib.h>
#include <stdarg.h>
// define MPFR_USE_VA_LIST to enable the use of mpfr_inits and mpfr_clears
#define MPFR_USE_VA_LIST
#include <mpfr.h>
#include "istring.h"
#include "array.h"
#include "math.h"
// add
Q Q_add(Q x1,Q x2){
Q ret;
ret.denominator=lcm(x1.denominator,x2.denominator);
ret.numerator=x1.numerator*(ret.denominator/x1.denominator)+x2.numerator*(ret.denominator/x2.denominator);
return(Q_simplify(ret));
}
//multiply
Q Q_prod(Q x1,Q x2){
return(Q_simplify(quot(x1.numerator*x2.numerator,x1.denominator*x2.denominator)));
}
// inverse
Q Q_inverse(Q x1){
if(x1.numerator>0){
return(quot(x1.denominator,x1.numerator));
}
else if(x1.numerator<0){
return(quot(-x1.denominator,-x1.numerator));
}
else{
fprintf(stderr,"error: attempting to invert %Lf/%Lf\n",x1.numerator, x1.denominator);
exit(-1);
}
}
// quotient
Q Q_quot(Q x1, Q x2){
if(x2.numerator>0){
return(Q_simplify(quot(x1.numerator*x2.denominator,x1.denominator*x2.numerator)));
}
else if(x2.numerator<0){
return(Q_simplify(quot(-x1.numerator*x2.denominator,-x1.denominator*x2.numerator)));
}
else{
fprintf(stderr,"error: attempting to invert %Lf/%Lf\n",x2.numerator, x2.denominator);
exit(-1);
}
}
// compare
int Q_cmp(Q x1, Q x2){
Q quo=Q_quot(x1,x2);
if(quo.numerator > quo.denominator){
return(1);
}
else if(quo.numerator < quo.denominator){
return(-1);
}
else{
return(0);
}
}
// simplify
Q Q_simplify(Q x){
return(quot(x.numerator/gcd(x.numerator,x.denominator),x.denominator/gcd(x.numerator,x.denominator)));
}
//simplify in place
int Q_simplify_inplace(Q* x){
Q ret=Q_simplify(*x);
*x=ret;
return(0);
}
// greatest common divisor
long double gcd(long double x, long double y){
long double ax=fabsl(x);
long double ay=fabsl(y);
int security=0;
while(ax!=0 && ay!=0){
if(ax>ay){ax=modld(ax,ay);}
else{ay=modld(ay,ax);}
security++;
if(security>1000000){
fprintf(stderr,"error: could not compute gcd( %Lf , %Lf ) after %d tries\n",x,y,security);
exit(-1);
}
}
// if both are negative, gcd should be negative (useful for simplification of fractions and product of square roots)
if(x<0 && y<0){
ax*=-1;
ay*=-1;
}
if(fabsl(ay)>fabsl(ax)){return(ay);}
else{return(ax);}
}
long double modld(long double x, long double m){
long double q=floorl(x/m);
return(x-q*m);
}
// least common multiple
long double lcm(long double x,long double y){
if(x!=0 || y!=0){
return((x*y)/gcd(x,y));
}
else{
return(0);
}
}
// approximate value as double
double Q_double_value(Q q){
return(1.0*q.numerator/q.denominator);
}
// approximate value as mpfr float
int Q_mpfr_value(mpfr_t out, Q q){
mpfr_t x;
mpfr_init(out);
mpfr_init(x);
mpfr_set_ld(x, q.denominator, MPFR_RNDN);
mpfr_ld_div(out, q.numerator, x, MPFR_RNDN);
mpfr_clear(x);
return(0);
}
// print to string
int Q_sprint(Q num, Char_Array* out){
if(num.denominator!=1){
char_array_snprintf(out,"%Lf/%Lf", num.numerator,num.denominator);
}
else{
char_array_snprintf(out,"%Lf",num.numerator);
}
return(0);
}
#define PP_NUMERATOR_MODE 1
#define PP_DENOMINATOR_MODE 2
// read from a string
int str_to_Q(char* str, Q* num){
char* ptr;
int mode;
char* buffer=calloc(str_len(str)+1,sizeof(char));
char* buffer_ptr=buffer;
*num=quot(0,1);
mode=PP_NUMERATOR_MODE;
for(ptr=str;*ptr!='\0';ptr++){
if(*ptr=='/'){
sscanf(buffer,"%Lf",&((*num).numerator));
buffer_ptr=buffer;
*buffer_ptr='\0';
mode=PP_DENOMINATOR_MODE;
}
else{
buffer_ptr=str_addchar(buffer_ptr,*ptr);
}
}
// last step
if(mode==PP_NUMERATOR_MODE){
sscanf(buffer,"%Lf",&((*num).numerator));
}
else if(mode==PP_DENOMINATOR_MODE){
sscanf(buffer,"%Lf",&((*num).denominator));
}
free(buffer);
return(0);
}
#else
int null_declaration_so_that_the_compiler_does_not_complain;
#endif
|